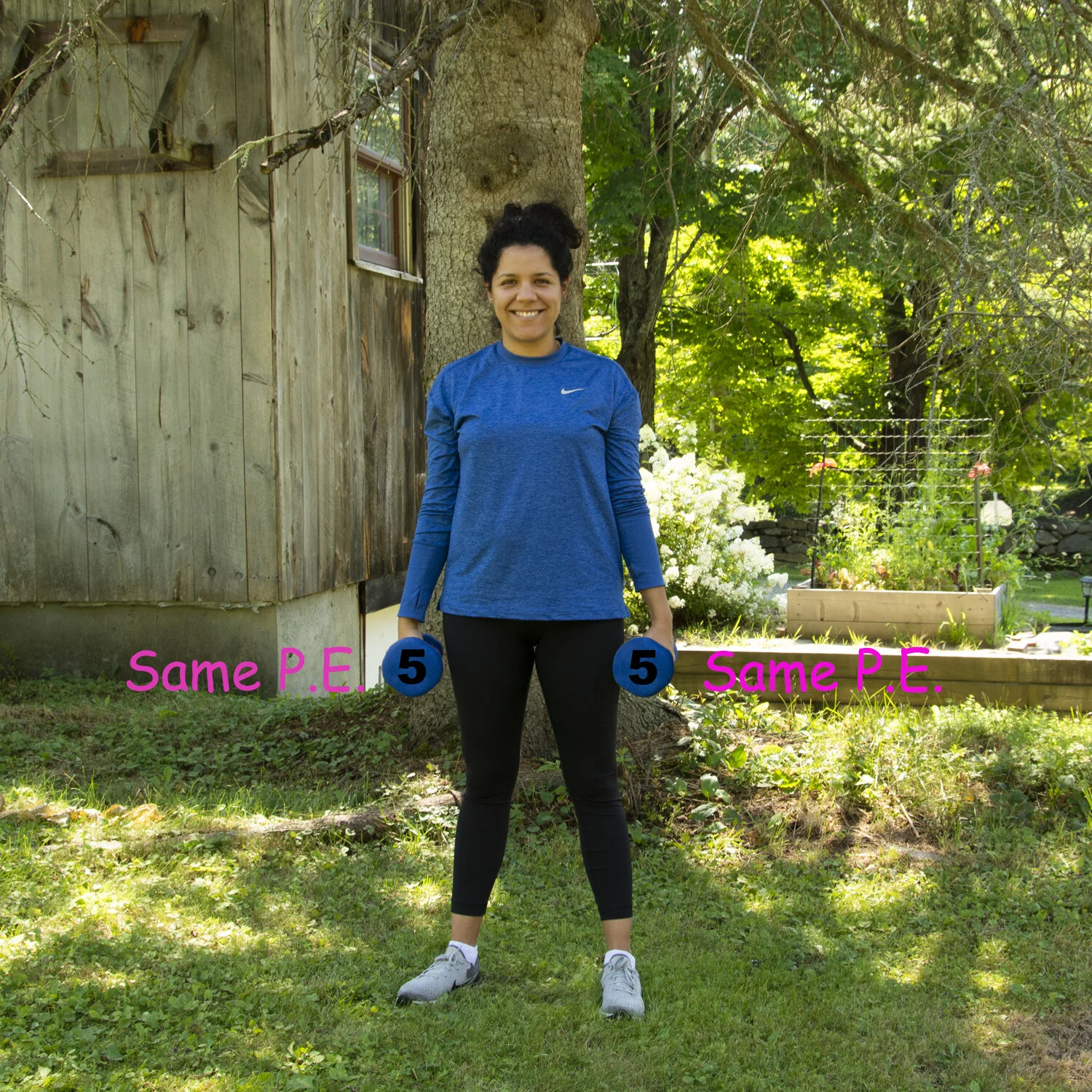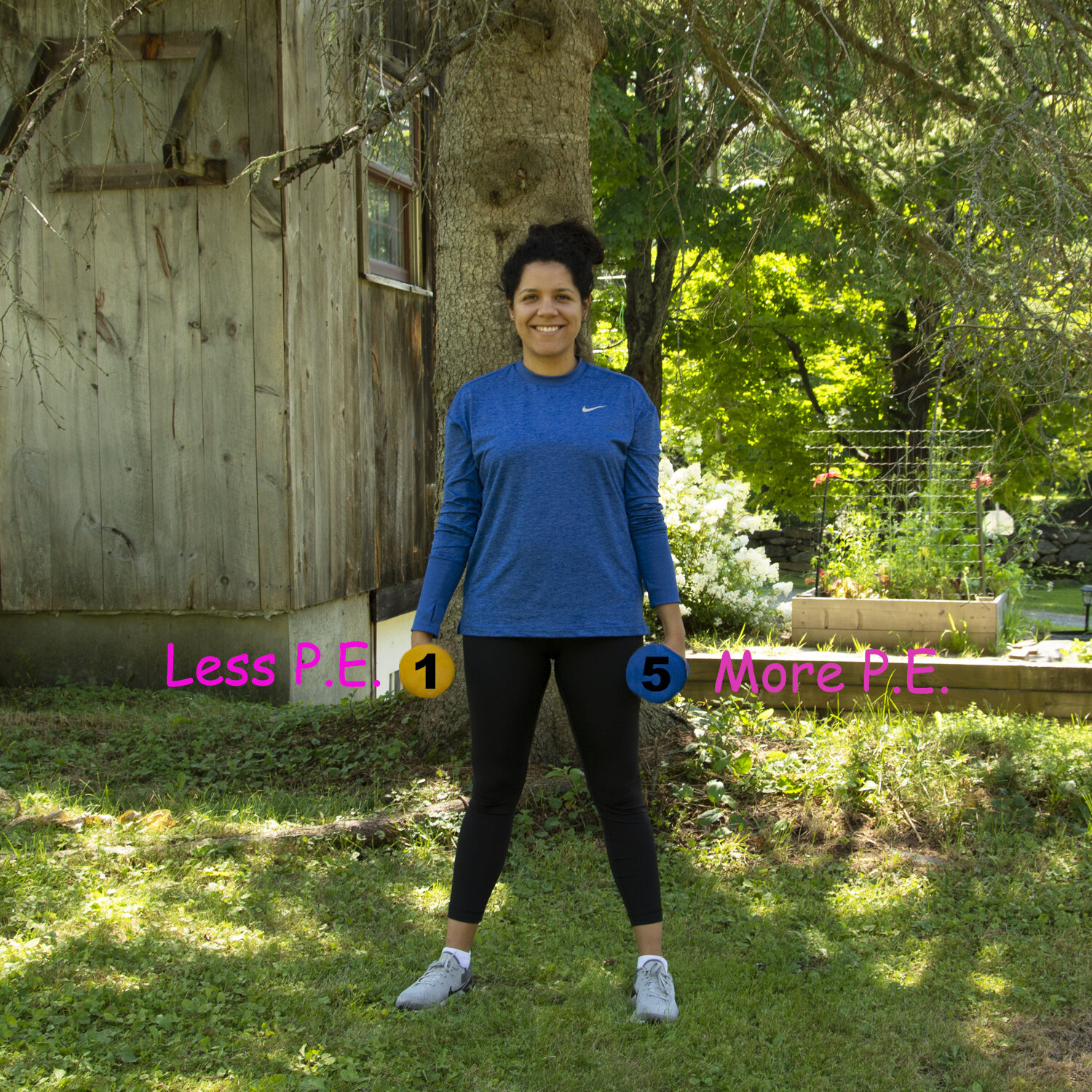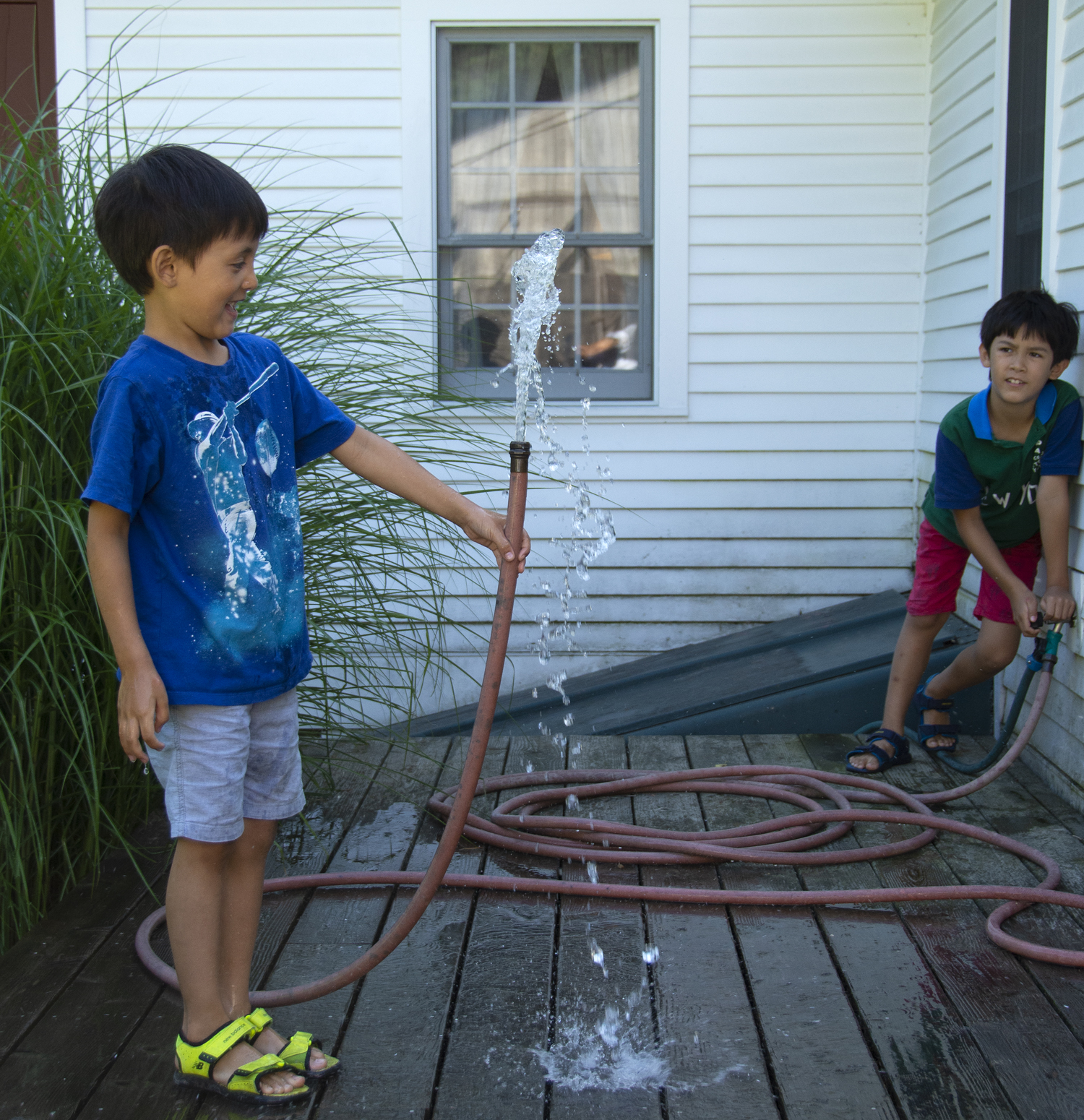Energy and Work
In order to understand swinging and bouncing, we need to first talk about energy. Energy is a word we hear all the time. You can’t see it and you can’t measure it with a ruler, a balance scale or a speedometer. We all know it’s there, though. You may feel like you have a lot of energy sometimes and not very much at other times. A person who is in constant motion is sometimes called energetic. We can look at that person and say that he or she has a lot of energy! But think about a weightlifter. A weightlifter might not be moving very much, but if she can lift a heavy barbell, she needs to have a lot of energy ready to use. Thus, one way to define energy is to say that if you have it, you have the ability to move something - like yourself or a weight or just a piece of paper.
Kinetic Energy
There are several types of energy. To describe any back and forth motion, two types are needed. The first is called kinetic energy. Kinetic energy only exists when something is moving. That means it is zero for any object that is sitting still. Balls flying through the air, people running, and turtles inching through the grass all have kinetic energy. Boulders sitting in a field do not.
The amount of kinetic energy a moving object has depends on two things: its mass and its velocity. Suppose a little car and a big truck are traveling along the road with the same velocity. If the truck has a mass that is ten times that of the car, its kinetic energy is also ten times that of the car. We saw this kind of comparison when we discussed momentum. If the mass of the car and truck is different by a factor of 10, so is the momentum.
A truck with 10 times the mass of a car has 10 times as much kinetic energy if they are traveling at the same speed.
The relationship between kinetic energy and velocity is different, however. Suppose two identical marbles are rolling on a table and one is going twice as fast as the other. It covers twice as much distance as the slower marble in the same period of time. The fast marble’s kinetic energy is 4 times that of the slow marble. In other words, if the velocity differs by a factor of 2, the kinetic energy differs by a factor of 4. This differs from momentum, where, for two identical marbles, one with twice the velocity of the other will have twice the momentum.
A marble rolling with twice the velocity of another marble with the same mass has 4 times the kinetic energy.
When we discussed momentum, we used an example of a car traveling 30 mph on the open road and 10 mph in traffic. When the car travels 30 mph, its kinetic energy is 9 times greater than when it travels 10 mph. In other words, if the velocity differs by a factor of 3, the kinetic energy differs by a factor of 9. If you have a knack for math, you will recognize this special relationship between kinetic energy and velocity: the kinetic energy depends on the velocity multiplied by itself, or squared. The momentum depends on the velocity.
There is another difference between momentum and energy. Recall that momentum is a vector, which means that it has a direction associated with it. Kinetic energy, in fact all energy, does not have a direction. In physics, things that don’t have any direction, like mass and now energy, are called scalars.
Potential Energy
The second kind of energy needed to explain back and forth motion is called potential energy. We can think of something having potential energy if it has the ability to make itself move. Unlike kinetic energy, an object can have potential energy if it is not moving. If you hold a ball above the ground, it has potential energy because it will move (start falling) if you let go. Once the ball starts to fall, it still has potential energy, which will cause it to continue moving until it hits the ground. It will also have kinetic energy, because it is moving.
There are many forms of potential energy. In the simple case of holding an object above the ground, the potential energy depends on two things: the mass of the object and its height above the ground. Suppose you hold two dumbbells at the same height above the ground. If they have the same mass, they have the same potential energy, as shown in the picture on the left below. However, if the mass of one dumbbell is five times the mass of the other one, the potential energy of the heavier dumbbell is five times that of the lighter one, as shown on the right.
We know that if you hold two identical dumbbells at the same height above the ground, they have the same potential energy. Now suppose you hold one dumbbell high above the ground and the other, identical one, lower to the ground. The potential energy of the high dumbbell is greater than that of the low one. If the high dumbbell is twice as high as the low one, its potential energy is twice as much as that of the low one.
What happens if there is a ball sitting on a table? There is no height difference between the table top and the ball, so the ball doesn’t have any potential energy relative to the table. Now suppose the ball rolls to the edge of the table and falls off. Just as it leaves the table, there is a difference in height between the ball and the floor so we see that the ball does have potential energy relative to the floor. When it comes to potential energy, the position of an object relative to its surroundings matters. This is true whether the object is a ball or a dumbbell or even a person!
The kind of potential energy we have just described is called gravitational potential energy because it depends on the gravitational pull of the earth. When we learn about springs, we will learn about another kind called spring potential energy.
Conservation of Energy
Kinetic and potential energy are two forms of mechanical energy. When they are added together, the sum is called the total mechanical energy. In an ideal world, the total mechanical energy is conserved whenever something happens. That means that the sum doesn’t change.
Consider again dropping a ball from some height above the ground. As soon as you let go, the ball has a lot of potential energy (because it is high off the ground) but very little kinetic energy (because it isn’t moving very fast). Once it starts to fall, it develops more kinetic energy owing to its increased velocity. At the same time, the potential energy reduces because the ball is getting closer to the ground. As the ball falls more, it goes even faster and gets even closer to the ground. This means that the kinetic energy continues to increase while the potential energy continues to decrease. Just before the ball hits the ground, it has only kinetic energy and that kinetic energy is equal to the potential energy that the ball had before it was dropped. In fact, everywhere along the path of the falling ball, the ball’s energy gets transferred from potential to kinetic while the total mechanical energy - kinetic plus potential – stays the same.
The total mechanical energy stays the same as the ball falls to the ground.
One way to think about conservation of energy is to have two glasses, one representing kinetic energy (K.E.) and the other representing potential energy (P.E.). Start with some water in the P.E. glass and think of the water as representing energy.
Energy conservation can be demonstrated by pouring water from a “potential energy” glass into a “kinetic energy” glass.
Slowly pour the water from the P.E. glass into the K.E. glass. The water slowly changes from being in the P.E. glass to being in the K.E. glass, just like the energy slowly transfers from potential to kinetic when you drop a ball. When you are done pouring, all of the water is in the K.E. glass. This is just like what happens to the ball before it hits the ground when it has only kinetic energy and no more potential energy. While you are pouring, the total amount of water stays the same but it is divided up differently between the two glasses. While the ball falls, the total mechanical energy stays the same but is divided up differently between kinetic and potential.
Energy is conserved if you start out with only kinetic energy and end up with only potential energy. At a splash pad, for example, water jets leave the ground and point upward. The water has only kinetic energy at ground level, but as it rises, gravity causes it to slow down. The kinetic energy reduces as some of it is converted to potential energy. At the top, the water stops moving before turning around and heading back to the ground. When it is stopped, it has only potential energy. As it falls back to the ground, that potential energy is converted back to kinetic energy. For all of the time that the water is in the air, the sum of the kinetic and potential energy stays the same.
At a splash pad, the kinetic energy of the water at the ground is converted to potential energy as it rises.
Another way to think about energy conservation is to think about the amount of energy that is transferred from one form to another. If you start with a small amount of K.E. (or P.E.) you will only get a small amount of P.E. (or K.E.) if the energy gets converted from one kind to the other. Suppose you hold onto a hose pointing up and your friend changes the amount of water coming out of the faucet at the other end. If the water comes out slowly, it doesn’t have much kinetic energy. When the water goes up, slows down and comes to a stop, it doesn’t go very far because there isn’t much kinetic energy to be converted to potential energy.
When your friend opens up the faucet more, the water comes out faster. It has more kinetic energy so the water jet goes higher before it turns around. At the top, it has more potential energy because it started with more kinetic energy. When the water comes out really fast, stand back because you might get wet!
Work
We all know what it means to do work! There are lots of ways we can do work: picking clothes up off the floor, cutting the grass, or doing math problems at a desk. But in physics, the term “work” has a very specific meaning and it doesn’t include all of the kinds of work we normally think of. In physics, work only happens when you exert a force over some distance. Remember that force and distance are both vectors. For work to be done, at least some part of the force (a component of the force, remember?) has to be pointing in the same direction as the distance over which the force is applied. If the force vector is perpendicular to the distance vector, no work is done!
Here are some examples. Can you tell if work is done for each of them?
When you lift a bucket, the net upward force is parallel to the distance moved by the bucket, so work is done. When you hold a bucket steady, there is a force acting (by your arm and hand) but since there is no motion, no work is done. When a tugboat pulls a tanker, the force applied by the towing line is in the same direction as the motion of the tanker, so work is done. When you are studying at your desk, there is no force and no motion so, sadly, you aren’t getting any work done!
It is helpful to talk about work when we talk about energy because energy is needed to do work. And if work is done, energy is created! Suppose you throw a ball into the air. When the ball is in your hand, you lift it upward for some distance. The force you apply is a little bit more than the weight of the ball, but in the opposite direction. It is the net force, F, shown in yellow below, that lifts the ball. Since the net force and the distance, d, also in yellow, are vectors that point in the same direction (up), you do work while the ball is in your hand. When you let go of the ball, it is moving. Its kinetic energy is due to the work you did to lift it before you let go.
When work is done, energy is created. In this example, kinetic energy is created because the ball is moving when you let go.
What happens next? We all know that the ball travels up some distance, then stops for an instant before changing direction and falling back toward the earth. On the way up, the kinetic energy decreases and the potential energy increases. At its maximum height, the ball has no more kinetic energy since it isn’t moving. Instead, all of its energy is in the form of potential energy.
The kinetic energy of the ball gradually changes to potential energy on the way up. The potential energy then gradually changes back to kinetic energy on the way down.
That potential energy soon gets converted back to kinetic energy as the ball falls. But this whole process begins with work being done on the ball, giving it some kinetic energy in the first place.
The transfer of energy from potential to kinetic – or from kinetic to potential – happens all the time. Swinging on a swing and bouncing on a trampoline are two great examples. To better understand how and when this transfer of energy takes place, we will take a look at springs. It is easy to think about something bouncing on the end of a hanging spring and in the next section, we will show why this bouncy behavior happens.